How do you defining and taking derivatives?
6 min read•july 11, 2024
Rupi Adhikary
AP Calculus AB/BC ♾️
279 resourcesSee Units
At this point, you should be able to recognize quite a few ways to write the derivative:
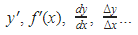
...and so on. However, on the AP exam, you need to know more than just how to write or take the derivative of a function. In this article, I'll be going over the definitions of the derivative and some common derivatives that you'll need to know for the AP exam.
Defining the Derivative
Both definitions of the derivative that you should know center around the idea of the derivative as the slope of the secant line passing between two points. To find the instantaneous rate of change (A.K.A, the derivative) for a spot on the graph between those two points, the points must grow infinitesimally closer together. Put another way, the space between them must approach 0 - effectively, the two points have now "become" the one point you are trying to find the derivative of.
The Limit Definition
This is the definition that most often appears on the multiple-choice and free-response sections of the AP exam, and you will either have to identify it out of other incorrect choices or use it to find a derivative. It is as follows:
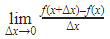
- Since the slope of the secant line between two points is the change in y divided by the change in x, the expression without the limit is simply the equation for the secant line: f(x) represents your "initial" y-value, and f(x+delta x) represents your "final" y-value.
- Once you add the limit to the expression, the formula's use as the definition of the derivative becomes clear: it is the slope of the secant line as the distance between the two points (delta x) approaches 0. Thus, this formula is used to approximate the instantaneous rate of change (the derivative) of a point on the graph.
- This definition may also be referred to as the difference quotient method
The Alternate Definition
The second definition of the derivative that you should be able to recognize is the alternate definition (I know, it's a really creative name). It looks like this:
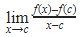
- This definition more clearly shows the equation of the secant line (change in y over change in x). By taking the limit as x approaches c, the two points are getting infinitesimally closer together and effectively "become" one point whose derivative you are approximating.
The limit definition appears much more often on the AP exam than the alternate definition, but you should still be able to recognize and use both of them.
Calculating Basic Derivatives
With taking a derivative, there are a couple of rules that you'll need to know
Power Rule
- The formula for the power rule looks something like this
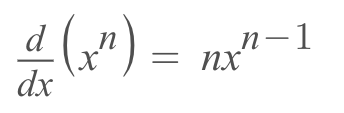
- With this formula, 'x' is the variable, and 'n' is the exponent
- First, you have to look at the exponent. It's also important to remember your exponent rules here too (anything to the power of 0 is 1, negative exponents, etc.)
- Next, you need to multiply the coefficient of the variable by the exponent
- The last step is to subtract one from the original exponent. This is now the new exponent for the derivative!
Let's go over a quick example then:

- We can see that the we have an exponent of two and a coefficient of 3.
- Now that we've noted this, we have to multiply the coefficient by the exponent.
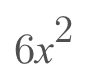
- Last but not least, we have to subtract one from the exponent value
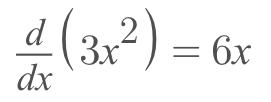
- And voila! You've taken a derivative using the power rule!
Sum and Difference Rule
This rule is pretty straight forward and is used when you're taken a derivative of a function that has multiple terms.
- The rule in its formal form looks like this
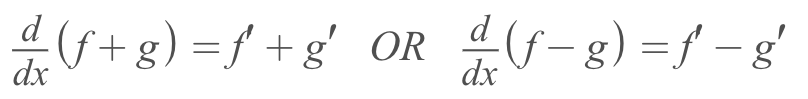
- The first one shows the sum rule while the second one shows the difference rule (That apostrophe means 'the derivative of')
- This one's a pretty easy one to pick up on so we're gonna go ahead and go into the next one! If you need additional practice with this one there'll be some links to some great resources for practice problems.
Product Rule
- This rule is used when two parts of a function are being multiplied by each other (this is different from chain rule!)
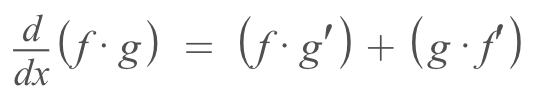
- This looks super confusing but the saying my teacher taught me really helped: "first times the derivative of the second plus second times the derivative of the first"
- In this case, the 'first' would be the variable 'f' while the 'second' is the variable is 'g'
- First, you'll need to identify your two variables, pay attention to their coefficients and exponents
- Next, identify which term will be your 'first' and which will be your 'second'
- Now you're going to take the 'first' and multiply it by the derivative of the second and vice versa.
This can get pretty confusing through just words so we'll go over a quick example to help see how it should be done!
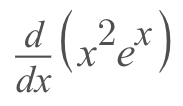
- This first step is to identify our terms. We have 'x^2' and 'e^x' and we can see that they are being multiplied by each other. Now, we'll assume that 'x^2' is going to be our 'first' and 'e^x' is going to be 'second'
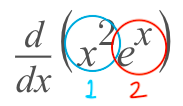
- Now, we have to follow through with the formula and saying: "first times the derivative of the second plus second times the derivative of the first"
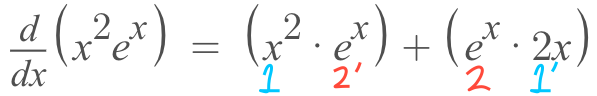
Quotient Rule
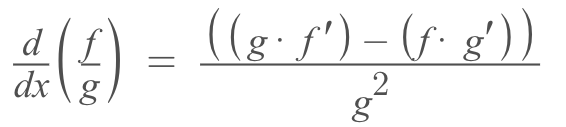
- Just like the product rule, this one's a little bit of a doozy and so my teacher taught us a song to help remember this (it's meant to go with the Snow White and the Seven Dwarfs HeIgh-Ho song): "You take the low, d hi, minus the high, d low, square the bottom and away we go, heigh-ho, heigh-ho!"

- This rule is more about identifying when you should use it and following the formula.
- With certain problems, especially since this is such a long process, you could change the denominator to a negative exponent (1/x = x^-1) and then use product rule
Chain Rule
- Last but not least, we have chain rule. This is definitely the one that most people have the hardest time with so it's important that you can get the basics of it first.
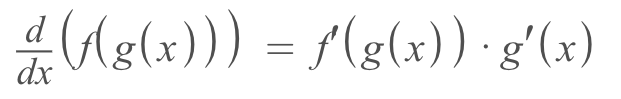
- This would sound something like "the derivative of f of g of x equals to the f prime of g of x times g prime of x"
- This is used when a function is composed of another one (kinda confusing, but it'll make sense)
- It's sometimes called the "outside inside rule", since it is the derivative of the outside ignoring the inside times the derivative of the inside
- Say you have a problem like this:
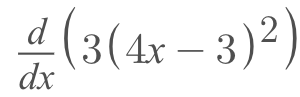
- First, we need to identify our inner and outer functions. If we treat what's in the inner parentheses as one function and replace the whole with the variable 'x' for now.
- What we have now is
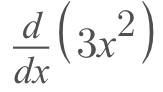
- We know how to take the derivative of this (power rule!) so we have the first part of this problem done
- Now, we have to take the derivative of the 'inside' part of the original function
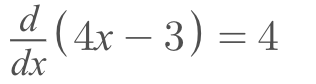
- The last step is to multiply these two parts together
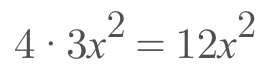
And that's it! These were the basic rules for taking a derivative that you'll need to know in order to get that five! If you need some additional practice, check out the resources linked below!
What Should I Memorize?
The chart below has the most common derivatives that appear on the exam that you should know. Remember, these are listed in their most basic forms, so be prepared to see them with negative signs and coefficients in front of them and adjust your derivative accordingly. You should also know how to use these derivatives in conjunction with other functions (such as when using the product rule, quotient rule, or chain rule). Finally, you may notice that these functions seem quite familiar. This is because they are all either parents functions or trig functions
to have memorized!
to have memorized!
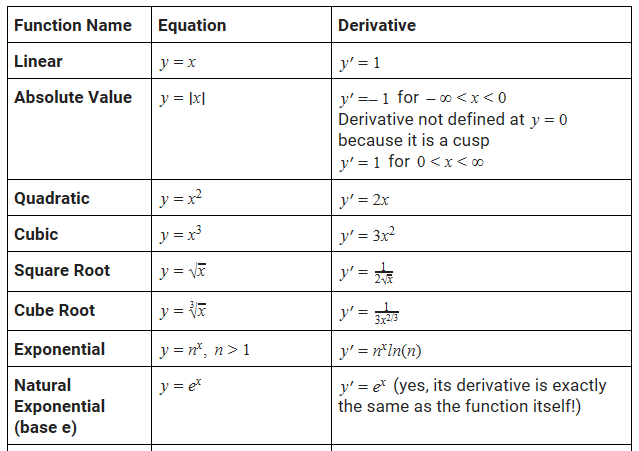
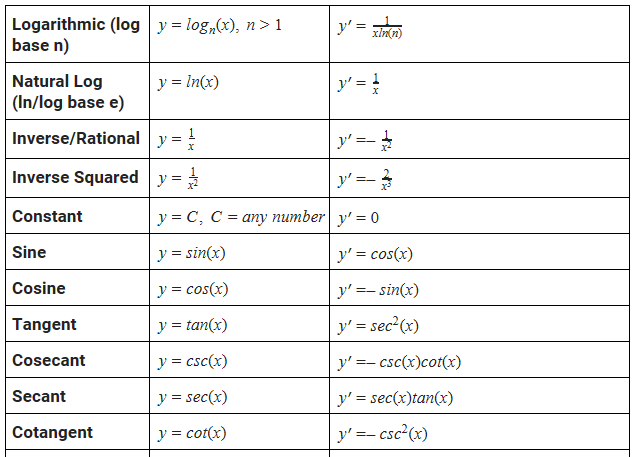
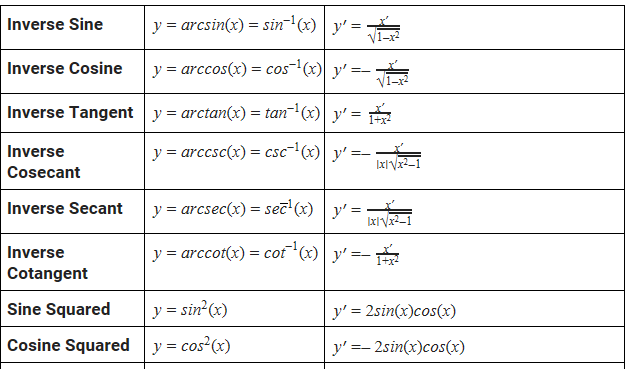
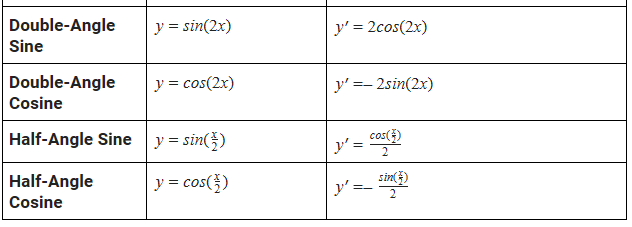
For the AP Calculus AB exam, it is not required to have these derivatives memorized, but it will certainly make solving problems where you need to take the derivative of a function a lot faster. Again, you should be able to take the derivatives of these functions with modifications (negative signs and coefficients) and using the product, quotient, or chain rules. If you're familiar with these common derivatives, rules, and definitions, your year in AP Calculus will be a breeze.
Browse Study Guides By Unit
👑Unit 1 – Limits & Continuity
🤓Unit 2 – Fundamentals of Differentiation
🤙🏽Unit 3 – Composite, Implicit, & Inverse Functions
👀Unit 4 – Contextual Applications of Differentiation
✨Unit 5 – Analytical Applications of Differentiation
🔥Unit 6 – Integration & Accumulation of Change
💎Unit 7 – Differential Equations
🐶Unit 8 – Applications of Integration
🦖Unit 9 – Parametric Equations, Polar Coordinates, & Vector-Valued Functions (BC Only)
♾Unit 10 – Infinite Sequences & Series (BC Only)
📚Study Tools
🤔Exam Skills

Fiveable
Resources
© 2025 Fiveable Inc. All rights reserved.