📚

All Subjects

🎡

AP Physics 1

👟

Unit 1

1.2 Representations of Motion

12 min read•june 18, 2024

Peter Apps

Daniella Garcia-Loos

AP Physics 1 🎡

257 resources

See Units

Understanding Representations of Motion

In AP Physics 1, we study different representations of motion to understand and analyze the movement of objects. Some common types of representations include:

Graphical representations: These include position-time graphs, velocity-time graphs, and acceleration-time graphs. These graphs can be used to represent the motion of an object and to understand its characteristics, such as its speed and acceleration.
Numerical representations: These include tables or lists of numerical data that describe the motion of an object. These data may include position, velocity, and acceleration at different times.
Analytical representations: These include mathematical equations that describe the motion of an object. These equations may involve variables such as position, velocity, and acceleration, and can be used to make predictions about the motion of an object.
Diagrammatic representations: These include sketches or diagrams that show the position, velocity, and acceleration of an object at different times. These representations can help visualize and understand the motion of an object.

Overall, different representations of motion can be used to help understand the characteristics and behavior of moving objects, and can be useful tools for predicting and analyzing the motion of objects in different situations.

Center of Mass

The acceleration of the center of mass of a system is related to the net force exerted on the system, where a = F/m.

Key Vocabulary: Center of mass - a point on an object or system that is the mean position of the matter.

⟶ A force may be applied to this point to cause a linear acceleration without angular acceleration occurring.

4.A.1 Essential Knowledge ➡️

The linear motion of a system can be described by the displacement, velocity, and acceleration of its center of mass.

4.A.2 Essential Knowledge ⏰

The acceleration is equal to the rate of change of velocity with time, and velocity is equal to the rate of change of position with time.

Graphical Representations of Motion 📈

As we covered in 1.1 Position, Velocity and Acceleration there are ways to represent all three quantities graphically. We also reviewed how to interpret these graphs, but it is imperative to understand the relationships each graph has to one another.

Image Courtesy of geogebra

From the image above, we can use position to find velocity for any given period of time by looking at the slope of the Position vs. Time Graph. Working from velocity to position we can look at the area underneath the curve to find displacement, but it is not possible to determine how far from the detector the object is located from a Velocity vs. Time Graph.

When working from velocity to acceleration we look at the slope of the Velocity vs. Time Graph. Similarly, when working from acceleration to velocity we look at the area under the curve to find velocity.

Linearization 🤸‍♀️

Many graphs in physics will not be perfectly straight lines, but we can turn the curve into a straight sloped line! We accomplish this by squaring the x-axis value. This is called Linearization. This is a concept that will be used throughout the course, so get comfortable with it now!

Image Courtesy of x-engineer.org

Linearization of a graph is a method of approximating the behavior of a nonlinear function with a straight line. This can be useful for making predictions or understanding the general trend of the function. Here are some key points to consider when linearizing a graph:

A linear function is defined by a straight line, which can be represented by the equation y = mx + b, where m is the slope and b is the y-intercept.
A nonlinear function is any function that is not a straight line. Nonlinear functions can take many different shapes, including curves, loops, and jumps.

To linearize a nonlinear function, we need to find a straight line that is a good approximation of the function. This can be done by finding two points on the nonlinear function and drawing a straight line through them.
The equation of the tangent line can be found using the point-slope form of a linear equation: y - y1 = m(x - x1), where (x1, y1) is a point on the function and m is the slope of the tangent line at that point.
The accuracy of the linear approximation depends on how closely the straight line follows the shape of the nonlinear function. The closer the line is to the function, the more accurate the approximation will be.
Linearization can be useful in physics because many physical systems can be modeled with nonlinear functions. By linearizing these functions, we can make predictions and understand the general trend of the system's behavior.

Example Problem

A ball is dropped from a height of 10 meters, and we want to find the time it takes to hit the ground. We know that the equation for the position of the ball as a function of time is:

y = 10 - 4.9t^2

However, this is a nonlinear equation and it is difficult to solve for t. Instead, we can use linearization to find an approximate solution.

To linearize the equation, we need to find the equation of the tangent line at a specific point on the curve. Let's choose the point where t = 1 second. At this point, the position of the ball is:

y = 10 - 4.9(1^2) = 5.1

Now we need to find the equation of the tangent line at this point. We can do this by using the point-slope form of a linear equation: y - y1 = m(x - x1), where (x1, y1) is the point on the curve and m is the slope of the tangent line at that point.

Since the point on the curve is (1, 5.1), we can set y1 = 5.1 and x1 = 1. To find the slope of the tangent line, we can plot a second point on the curve that is close to (1, 5.1) and find the slope between the two points. For example, we can choose the point where t = 1.1 seconds:

y = 10 - 4.9(1.1^2) = 4.61

The slope between the two points is (4.61 - 5.1)/(1.1 - 1) = -0.49.

Now we can plug the values into the point-slope form of the linear equation to find the equation of the tangent line:

y - 5.1 = -0.49(x - 1)

y = -0.49x + 5.6

We can use this linear equation to approximate the position of the ball as it falls. For example, if we want to find the position of the ball after 0.5 seconds, we can substitute t = 0.5 into the linear equation to get:

y = -0.49*0.5 + 5.6 = 5.355

This means that the ball has fallen about 5.355 meters after 0.5 seconds.

This is just an approximate solution, but it is a much simpler calculation than solving the nonlinear equation for the position of the ball. Linearization can be useful for finding approximate solutions to problems involving nonlinear functions, especially when the nonlinear function is difficult to work with or when we only need an approximate solution.

⟶ Still feeling a little confused on Linearization? Don’t worry! Check out this video from AP Physics 1 Online for more practice!

Mathematical Representations of Motion 📍

In Kinematics there are four major equations you must understand to begin calculations. They relate acceleration, displacement, initial and final velocity, and time together.

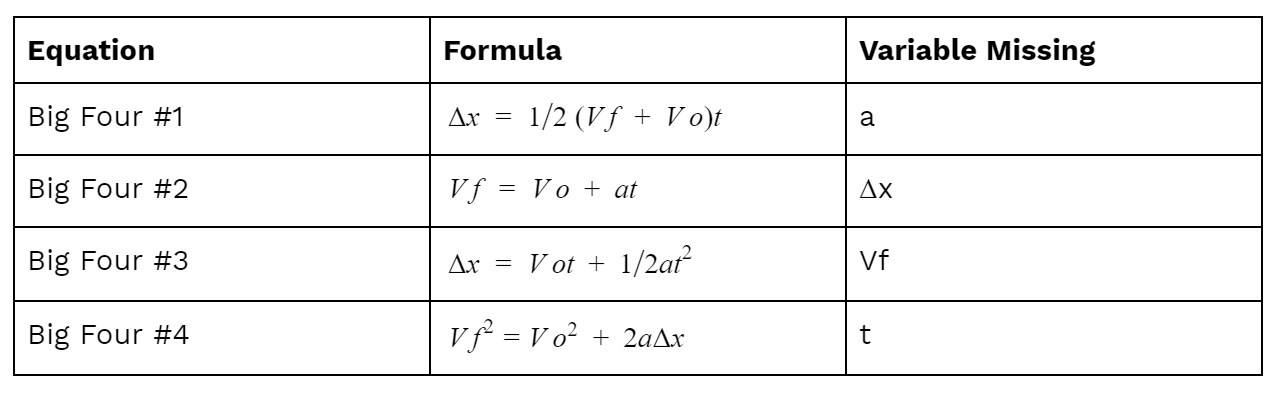
Variable Interpretation: Δx is horizontal displacement in meters, Vf is final velocity in meters/second, Vo is initial velocity in meters/second, t is time in seconds, and a is acceleration in m/s/s.

⟶ In order to solve for a variable without having all four other quantities known, we look at the ‘Variable Missing’ column to pick the equation that best suits our question.

EXAMPLE:

A super car races by at a speed of 68 m/s and slows down as a rate of 4 m/s/s.

How much runway is needed to stop the plane?

We are given the variables a, Vo, and Vf but we are missing Δx and t

Vf^2 =Vo^2 + 2aΔx

STEP 1: Cross out Vf because the car will stop at a velocity of 0 m/s
STEP 2: Plug in the known variables: 0 = (68 m/s^2) + 2 (-4 m/s/s^2) (Δx)

Your final answer should be Δx = 578 m

Free Fall ⚽️

Key Vocabulary: Free Fall - an object only under the influence of gravity

Equation: velocity = force of gravity x time

Key Vocabulary: Acceleration due to Gravity - 9.8 m/s/s (it is acceptable to round up to 10 m/s/s on the AP Physics 1 exam)

Equation	Formula	Variable Missing
Big Four #2	Vf = Vo + gt	Δy
Big Four #3	y = Vot + 1/2 gt2	Vf
Big Four #4	Vf2 = Vo2 + 2gy	t

Variable Interpretation: Δy is vertical displacement in meters, Vf is final velocity in meters/second, Vo is initial velocity in meters/second, t is time in seconds, and g is acceleration due to gravity in m/s/s.

⟶ In free fall equations, we now replace Δx with Δy and a with g giving us a modified list of The Big Four as seen in the table above.

Object Dropped (trip down)

Vo (initial velocity) = 0 m/s
g works in the direction of motion

Object Tossed (trip up)

Vf (final velocity) = 0 m/s

At maximum height of its trip, an object has a velocity of 0 m/s

g works against the direction of motion

EXAMPLE #1:

A ball is dropped from the top of a building. It falls 2.8s.

What is the displacement of the ball?

We are given the variables g, t, and Vo, but we are missing Δy and Vf

Δy = Vot + ½ gt2

STEP 1: Cross out Vo because an object that is dropped has an initial velocity of 0 m/s
STEP 2: Plug in the known variables: Δy = ½ (10 m/s/s) (2.8 s2)

Your final answer should be Δy = 39.2 m

What is the ball’s final velocity?

We are given the variables g, t, and Vo, but we are missing Δy and Vf

Vf = Vo + gt

STEP 1: Cross out Vo because an object that is dropped has an initial velocity of 0 m/s
STEP 2: Plug in the known variables: Vf = (10 m/s/s) (2.8 s)

Your final answer should be Vf = 28 m/s

EXAMPLE #2:

A soccer ball is thrown straight up with an initial velocity of 20 m/s.

What height did the soccer ball reach?

We are given the variables g, Vo, and Vf, but we are missing Δy and t

Vf2 = Vo2 + 2gΔy

STEP 1: Cross out Vf because an object thrown up has a final velocity of 0 m/s
STEP 2: Plug in known variables: 0 = (20 m/s2) + 2(10 m/s/s) (Δy)

Your final answer should be Δy = 20 m

Projectile Motion ☄️

Projectile motion is the motion of an object that is thrown, launched, or projected into the air and is then subject only to the force of gravity. Here are some key points to consider when solving projectile motion problems:

In projectile motion, the object moves in two dimensions: horizontally and vertically.
- The horizontal motion of a projectile is uniform, which means it moves at a constant speed in a straight line. This is because there are no forces acting on the projectile in the horizontal direction.
- The vertical motion of a projectile is affected by the force of gravity, which pulls the projectile downward. The force of gravity causes the projectile to accelerate downward at a rate of 9.8 m/s^2.
The path of a projectile is called its trajectory, and it is a parabolic curve.
The initial velocity of a projectile is a vector that specifies both the magnitude and direction of the projectile's motion. It can be broken down into its horizontal and vertical components.
The range of a projectile is the horizontal distance it travels from the starting point to where it lands.
The maximum height of a projectile is the highest point it reaches during its motion.
The time of flight of a projectile is the total time it takes to complete its motion from the starting point to the landing point.
The angle at which a projectile is launched has an effect on its range, maximum height, and time of flight.
Air resistance is ignored

When dealing with horizontal projectile launches, launches that have an entirely horizontal initial velocity (ex. a ball rolling off a table), we break it up into two sets of equations: vertical and horizontal.

Formula	Type	Variable Missing
y = Voyt + 1/2 gt2	Vertical	Vfy
Vfy = Voy + gt	Vertical	Δy
Vfy2 = Voy2 + 2gy	Vertical	t
x = Vxt	Horizontal	N/A

Variable Interpretation: Δy is vertical displacement in meters, Δx is horizontal displacement in meters, Vfy is vertical final velocity in meters/second, Voy is vertical initial velocity in meters/second, Vx is horizontal velocity in m/s, t is time in seconds, and g is acceleration due to gravity in m/s/s.

As shown in the chart above, there are three equations we use for the vertical component of launches and one for the horizontal. You cannot put an x-component into a formula without a y-component!

EXAMPLE:

A tennis ball is rolling on a ledge with a velocity of 5 m/s. If the tennis ball rolls off the table, which is 1.5m high:

(a) How long would it take to hit the ground?

We are given variables Δy, Voy and Vx, but we are missing t, Δx, and Vfy

Δy = Voyt + ½ gt2

STEP 1: Cross out Voy because there is no initial vertical velocity
STEP 2: Plug in known variables: 1.5m = ½ (10 m/s/s) t2

Your final answer should be 0.55 seconds

(b) What will be the distance it travels before reaching the ground?

We are given variables Δy, Voy and Vx, but we are missing Δx and Vfy

Δx = Vxt

STEP 1: Plug in known variables: Δx = (5 m/s) (0.55 s)

Your final answer should be 2.75 meters

We are given variables Δy, Voy and Vx, but we are missing Vfy

Vfy = Voy + gt OR Vfy2 = Voy2 + 2gΔy

STEP 1: Plug in known variables: Vfy = (10 m/s/s) (0.55 s) OR Vfy2 = 2 (10 m/s/s) (1.5 m)

Your final answer should be 5.5 m/s

⟶ Still feeling a little confused on Projectile Launches? Don’t worry! Check out this live stream from Fiveable for more practice!

Angled Motion 🏹

Key Vocabulary: Angled Launches - launches at an angle that includes both a horizontal and vertical component of initial velocity

Key Vocabulary: Vector Components - the horizontal and vertical parts of a vector

Angled launches require you to find the Vox and Voy, or vector components, based on the initial velocity Vo and the angle Ө. Now you can solve for the following: Vo, Vox, Voy, Ө, t, X, Ymax, and Vf.

If an object is shooting upward, Voy is (+)
If an object is shooting downward, Voy is (-)
Vertical velocity is 0 m/s at the top
Flight is symmetric if the projectile starts and ends at the same height

Angled Launch Formulas

cos(Vo) = Vox/Ө

sin(Vo) = Voy/Ө

Vox = Vocos(Ө)

Voy = Vosin(Ө)

t = 2(Voy)/g(This only applies if the starting and ending heights are the same)

Variable Interpretation: Voy is vertical initial velocity in meters/second, Vox is initial horizontal velocity in m/s, t is time in seconds, t is time in seconds, and g is acceleration due to gravity in m/s/s.

EXAMPLE:

A cannonball is shot at a 30 degree angle above the horizontal at 20 m/s.

(a) How much time will the cannonball travel for in the air?

We are given variables Ө and Vo, but we are missing t, Vox, and Voy

t = 2(Voy)/g AND Voy = Vosin(Ө)

STEP 1: Solve for Voy: Voy = 20sin(30), Voy = 10 m/s
STEP 2: Plug in known variables: t = 2(10 m/s)/(10 m/s/s)

Your final answer should be 2 seconds

(b) How far will the cannonball travel?

We are given variables Ө and Vo, but we are missing Vox

Δx = Vxt

STEP 1: Solve for Vx: Vox = 20cos(30), Vox = 17.3 m/s
STEP 2: Plug in known variables: Δx = (17.3 m/s)(2 s)

Your final answer should be 34.6 meters

We are given variables Ө, Vfy, and Vo, but we are missing Vox

Vfy2 = Voy2 + 2gΔy

STEP 1: Plug in known variables: 0 = (10 m/s)2 + 2(10 m/s/s)Δy

Your final answer should be 5 meters

⟶ Still feeling a little confused on Angled Launches? Don’t worry! Check out this video from Khan Academy for more practice! Want more practice - Check out the Fiveable Live streams on this topic: