4.6 Independent Events and Unions of Events
5 min read•june 18, 2024
Kanya Shah
Jed Quiaoit
AP Statistics 📊
265 resourcesSee Units
Independence is an important concept in probability because it allows us to simplify calculations by assuming that events are not affected by each other. When two events are independent, the probability of one event occurring does not depend on whether the other event has occurred or not.
For example, if you flip two coins, the likelihood of one landing on heads is not affected by the other coin. Therefore, we would say that these two events are independent. 🪙
On the other hand, if the temperature is extremely low, the probability of it snowing will increase. Therefore, these two events are not independent, or dependent, since the temperature does affect the likelihood of snow.
💡 DEFINITION: Events A and B are independent if, and only if, knowing whether event A has occurred (or will occur) does not change the probability that event B will occur.
Quantifying Independence
If two events are independent, then we can use the multiplication rule to find the probability of both events occurring. The multiplication rule states that the probability of two independent events occurring is the product of the probabilities of the individual events. This is written as P(A and B) = P(A) * P(B).
Another thing to keep in mind: if two events are independent, then the probability of one event occurring does not depend on whether the other event has occurred or not. This means that the probability of event A occurring (P(A)) is the same regardless of whether event B has occurred or not. Similarly, the probability of event B occurring (P(B)) is the same regardless of whether event A has occurred or not. 🌈
Therefore, if, and only if, events A and B are independent, then P(A | B) = P(A) and P(B | A) = P(B).
In other words, the probability of event A occurring (P(A)) is the same as the probability of event A occurring given that event B has occurred (P(A | B)). Similarly, the probability of event B occurring (P(B)) is the same as the probability of event B occurring given that event A has occurred (P(B | A)).
Unions & The Addition Rule
In a previous section, you were briefly introduced to unions, the probability that event A or event B (or both) will occur. Unions are often denoted as P(A ∪ B). From here, we can develop a new rule of finding probabilities for independent events. 👷
The addition rule is a way to find the probability of two events occurring together. It is also known as the "union" rule because it helps us find the probability of the union of two events.
The addition rule states that the probability of event A or event B occurring (denoted as P(A or B)) is equal to the probability of event A occurring (P(A)) plus the probability of event B occurring (P(B)) minus the probability of both events A and B occurring (P(A and B)). This is written as P(A or B) = P(A) + P(B) - P(A and B).
The addition rule applies when two events are not mutually exclusive (in other words, independent). This means that the events can occur at the same time and have some outcomes in common. In this case, we need to subtract the probability of both events occurring to avoid overcounting the common outcomes.
To keep the confusion at bay, here's a glimpse of the rules we've encountered so far, neatly organized in a visual:
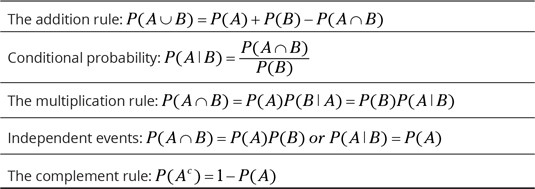
Source: Dummies
Example
Imagine you are studying the attendance at a local music festival. You want to find the probability that a person who attends the festival will visit at least one of the two main stages. The main stage has a capacity of 10,000 people and is expected to be 75% full on average. The second stage has a capacity of 5,000 people and is expected to be 50% full on average. 🎊
You know that the attendance at the two stages is independent, so you can use the union rule to find the probability that a person will attend at least one of the stages. The probability that a person will attend the main stage is 0.75 and the probability that a person will attend the second stage is 0.50. Using the union rule, you can calculate the probability that a person will attend at least one of the stages:
P(attend main stage or attend second stage) = P(attend main stage) + P(attend second stage) - P(attend main stage and attend second stage)
= 0.75 + 0.50 - (0.75 * 0.50)
= 0.75 + 0.50 - 0.375
= 1.25 - 0.375
= 0.875
The probability that a person will attend at least one of the stages is 0.875, or 87.5%.
Now, let's say you also want to find the probability that a person who attends the festival will attend both stages. In this case, the attendance at the two stages is not independent because a person who attends one stage is more likely to attend the other stage as well. Therefore, you cannot use the union rule to find the probability of both stages being attended. Instead, you can use the multiplication rule to find the probability of both events occurring:
P(attend main stage and attend second stage) = P(attend main stage) * P(attend second stage given that they attend main stage)
= 0.75 * 0.50
= 0.375
The probability that a person will attend both stages is 0.375, or 37.5%.
Overall, the probability that a person will attend at least one of the stages is higher than the probability that they will attend both stages, which makes sense because attending one stage does not necessarily mean that they will also attend the other stage. Understanding the union rule and the multiplication rule from previous sections can help you calculate the probability of events occurring together in different situations.
Practice Problem
Imagine you are studying the likelihood of getting a high score on a math exam. You know that the probability of getting a high score on the exam is 0.7 if you study for at least 20 hours, and 0.4 if you do not study for at least 20 hours. You also know that the probability of studying for at least 20 hours is 0.6. 😴
Find the probability of getting a high score on the exam regardless of whether you study for at least 20 hours or not.
Answer
To do this, you can use the union rule to find the probability of either event occurring:
P(high score | study for at least 20 hours or do not study for at least 20 hours) = P(high score | study for at least 20 hours) + P(high score | do not study for at least 20 hours) - P(high score | study for at least 20 hours AND do not study for at least 20 hours)
= 0.7 + 0.4 - (0.7 * 0.4)
= 0.7 + 0.4 - 0.28
= 1.1 - 0.28
= 0.82
The probability of getting a high score on the exam is 0.82, or 82%, regardless of whether you study for at least 20 hours or not.
Browse Study Guides By Unit
👆Unit 1 – Exploring One-Variable Data
✌️Unit 2 – Exploring Two-Variable Data
🔎Unit 3 – Collecting Data
🎲Unit 4 – Probability, Random Variables, & Probability Distributions
📊Unit 5 – Sampling Distributions
⚖️Unit 6 – Proportions
😼Unit 7 – Means
✳️Unit 8 – Chi-Squares
📈Unit 9 – Slopes
✏️Frequently Asked Questions
📚Study Tools
🤔Exam Skills

Fiveable
Resources
© 2025 Fiveable Inc. All rights reserved.