5.2 The Normal Distribution, Revisited
5 min read•january 13, 2023
Jed Quiaoit
B
Brianna Bukowski
Josh Argo
AP Statistics 📊
265 resourcesSee Units
The normal model is a continuous probability distribution that is characterized by a bell-shaped curve that is symmetrical around the mean. The mean, median, and mode of a normal distribution are all equal, and the distribution is unimodal, meaning that it has only one peak or mound. 🐪
To describe a normal model, you can talk about the center, shape, and spread of the distribution. The center of a normal model is represented by the mean, which is the arithmetic average of the data. The shape of a normal model is characterized by the bell-shaped curve, which is symmetrical around the mean. The spread of a normal model is represented by the standard deviation, which measures the dispersion or spread of the data around the mean.
In general, a model (such as a sampling distribution) is approximately normal if the following conditions are met for the following types of data:
Categorical (Proportions) | Quantitative (Means) |
|
OR
|
Empirical Rule Review
The area underneath any density curve (including the normal curve) is equal to 1, or 100% of the data. There is 50% of the data on each size of the mean.
- 68% of the data is within 1 standard deviation of the mean (34% on each side). 🔴
- 95% of the data is within 2 standard deviations of the mean (an additional 13.5% on each side). 🟣
- 99.7% of the data is within 3 standard deviations of the mean (an additional 2.35% on each side). 🟢
Source: Medium
🎥 Watch: AP Stats - Normal Distributions
A z-score indicates how many standard deviations above or below the mean a piece of data is. If a z-score is -2, it is 2 standard deviations below the mean. If a z-score is 1, it is 1 standard deviation above the mean. When asked to interpret a z-score, it is imperative that you indicate the direction (positive or negative). The magnitude of the z-scores is also important, especially when making inferences.
Z-scores are often used to compare scores regarding two sets of data, like SAT and ACT scores. The z-scores are resistant to units. Therefore the units do not matter and the z-scores remain the same if we convert the units. Think of z-scores as a way of comparing apples (🍎) to oranges (🍊).
Interpreting Percentiles in Normal Distributions
In a normal distribution, you can determine the intervals associated with a given area by assigning appropriate inequalities to the boundaries of the intervals.
For example, if you want to find the interval associated with an area of 0.95 (or 95%) under the normal curve, you would use the z-score values that correspond to that area. The z-score is a measure of how many standard deviations a value is from the mean of the distribution.
To find the z-scores that correspond to an area of 0.95, you can use a table of the standard normal distribution or a calculator or software program that can calculate them for you. The z-scores will be the boundaries of the interval, and you can use them to determine the values that fall within that interval by plugging them back into the equation for the normal distribution.
For example, if the mean of the distribution is 100 and the standard deviation is 15, the interval associated with an area of 0.95 would be between the values obtained by plugging the corresponding z-scores into the equation for the normal distribution.
We can also use actual equalities, as shown in the visual below:
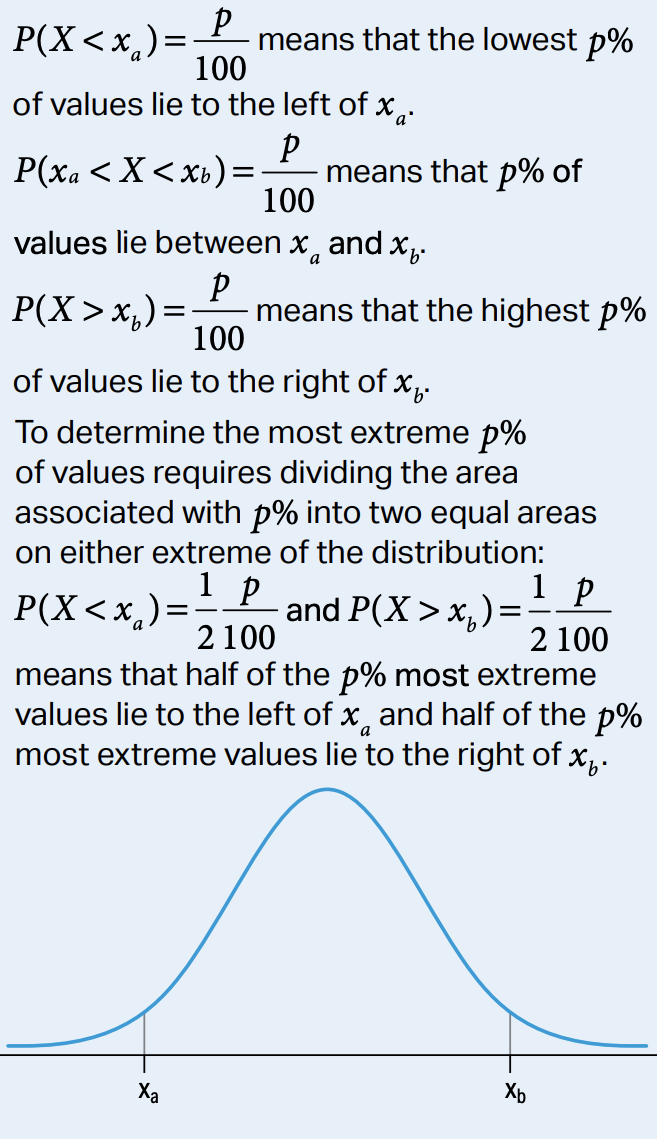
Source: College Board
Practice Problems
(1) A study is conducted to compare the heights of men and women in a certain population. The mean height of men is 70 inches, with a standard deviation of 3 inches. The mean height of women is 65 inches, with a standard deviation of 2.5 inches. Calculate the z-score for a man who is 72 inches tall.
(2) A study is conducted to compare the GPA of students at two different colleges. The mean GPA of students at College A is 3.0, with a standard deviation of 0.5. The mean GPA of students at College B is 2.5, with a standard deviation of 0.4. Calculate the z-score for a student at College A who has a GPA of 3.5.
(3) A study is conducted to compare the number of hours spent studying per week by students at two different universities. The mean number of hours spent studying per week at University A is 15 hours, with a standard deviation of 3 hours. The mean number of hours spent studying per week at University B is 12 hours, with a standard deviation of 2 hours. Calculate the z-score for a student at University A who studies 21 hours per week.
(4) A study is conducted to compare the heights of men and women in a certain population. The mean height of men is 70 inches, with a standard deviation of 3 inches. The mean height of women is 65 inches, with a standard deviation of 2.5 inches. Calculate the z-score for a woman who is 62 inches tall.
(5) Going back to problem (2), calculate the z-score for a student at College B who has a GPA of 3.0.
Answers
(1) To calculate the z-score for a man who is 72 inches tall, we can use the following formula:
z = (x - mean) / standard deviation
Plugging in the values, we get:
z = (72 - 70) / 3 = 2 / 3 = 0.67
Thus, the z-score for a man who is 72 inches tall is 0.67.
(2) Plugging in the values, we get:
z = (3.5 - 3.0) / 0.5 = 0.5 / 0.5 = 1
Thus, the z-score for a student at College A who has a GPA of 3.5 is 1.
(3) Plugging in the values, we get:
z = (21 - 15) / 3 = 3 / 3 = 2
Thus, the z-score for a student at University A who studies 18 hours per week is 2.
(4) Plugging in the values, we get:
z = (62 - 65) / 2.5 = -3 / 2.5 = -1.2
Thus, the z-score for a woman who is 62 inches tall is -1.2.
(5) Plugging in the values, we get:
z = (3.0 - 2.5) / 0.4 = 0.5 / 0.4 = 1.25
Thus, the z-score for a student at College B who has a GPA of 3.0 is 1.25.
Browse Study Guides By Unit
👆Unit 1 – Exploring One-Variable Data
✌️Unit 2 – Exploring Two-Variable Data
🔎Unit 3 – Collecting Data
🎲Unit 4 – Probability, Random Variables, & Probability Distributions
📊Unit 5 – Sampling Distributions
⚖️Unit 6 – Proportions
😼Unit 7 – Means
✳️Unit 8 – Chi-Squares
📈Unit 9 – Slopes
✏️Frequently Asked Questions
✍️Free Response Questions (FRQs)
📆Big Reviews: Finals & Exam Prep

Fiveable
Resources
© 2023 Fiveable Inc. All rights reserved.