2.3 Contact Forces

Types of Contact Forces ⛓

1. Friction: This is a force that opposes the motion of an object when it is in contact with a surface. Friction is what allows us to walk on the ground, hold onto objects, and drive cars.
2. Normal force: This is a force that acts perpendicular to a surface and supports the weight of an object resting on that surface. For example, when you sit on a chair, the chair exerts an upward normal force on you to support your weight.
3. Tension: This is a force that acts along a rope or wire and is used to transmit a pulling force from one object to another. For example, when you pull on a rope to lift a heavy object, the rope exerts a tension force on the object.

• Tension - The direction the rope, string, or chain is attached
• Friction - opposes the direction of motion
• Normal - Perpendicular to the surface
• Spring - Opposes the direction of the extension or compression
  

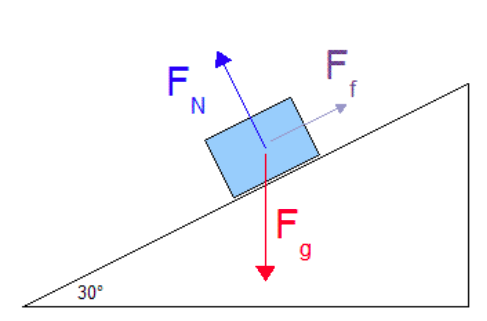
Image Courtesy of studyphysics.ca

• A normal force is a force that acts perpendicular to a surface and supports the weight of an object resting on that surface.
• The normal force arises due to the interaction between the particles that make up the object and the surface it is in contact with.
• The normal force is always present when an object is resting on a surface.

• The normal force is important because it determines the amount of other forces that can act on an object. For example, the normal force determines the amount of friction that can act on an object.

To solve this problem, we can use the equation for the normal force, which is given by Fn = Fgcosθ, where Fn is the normal force, Fg is the gravitational force, and θ is the angle of the ramp. The gravitational force is given by the equation Fg = mg, where Fg is the weight and g is the acceleration due to gravity.

Plugging in the given values, we have: Fg = (10.0 kg)(9.80 m/s^2) = 98.0 N

The normal force is given by the equation Fn = Fgcosθ, so: Fn = (98.0 N)cos(30°) = 86.6 N

Therefore, the normal force acting on the box is 86.6 N.

• Where k is the spring constant in units of Newtons per Meter (N/m) and x is the stretching or elongation of the spring beyond its original length. 

• Static friction is the friction that acts on an object when it is not moving, or when it is in a state of static equilibrium.
• Kinetic friction is the friction that acts on an object when it is moving.
• Static friction acts to prevent an object from moving, while kinetic friction acts to oppose the motion of an object.
• Static friction is generally larger than kinetic friction. This means that it takes more force to start an object moving from rest than it does to keep it moving at a constant speed.

• Static friction is always present when an object is resting on a surface. Kinetic friction is always present when an object is moving on a surface.
• The amount of static friction and kinetic friction depends on the materials of the two surfaces in contact.

To solve this problem, we need to use the equation of motion F = ma, where F is the total force acting on the object, m is the mass of the object, and a is the acceleration. In this case, the total force acting on the block is the sum of the gravitational force, the normal force, and the force of friction.

The gravitational force is given by the equation Fg = mg, where Fg is the weight and g is the acceleration due to gravity. Plugging in the given values, we have:

Fg = (4.00 kg)(9.81 m/s^2) = 39.24 N

The normal force is given by the equation Fn = Fgcosθ, where Fn is the normal force, Fg is the gravitational force, and θ is the angle of the ramp. Plugging in the given values, we have:

Fn = (39.2 N)cos(30°) = 33.97 N

The force of friction is given by the equation Ff = μFn, where Ff is the force of friction, μ is the coefficient of friction, and Fn is the normal force. Plugging in the given values, we have:

Ff = (0.400)(33.97 N) = 13.59 N Parallel Component: this helps the block to slide down the ramp. This is calculated as: F(parallel) =mg sin(30°) = 4.00 x 9.81 x sin(30) = 19.62N The net force along the incline is the driving force for the block's acceleration:

F(net) = F(parallel) - F(f) = 19.62 - 13.59 = 6.03 N

Now, we can use the equation of motion F = ma to calculate the acceleration of the block:

a = F/m = (6.03 N)/(4.00 kg) = 1.51m/s^2

Therefore, the acceleration of the block as it slides down the ramp is 1.51 m/s^2.