7.6 Properties of the Equilibrium Constant

In Unit 6 we discussed Hess’s Law and how it can be used to find the value of ΔH for reactions by adding together, flipping, and multiplying reactions. In this section, we’ll apply similar rules to equilibrium! If you did well with Hess’s Law problems then you should do fine with problems involving the properties of the equilibrium constant.

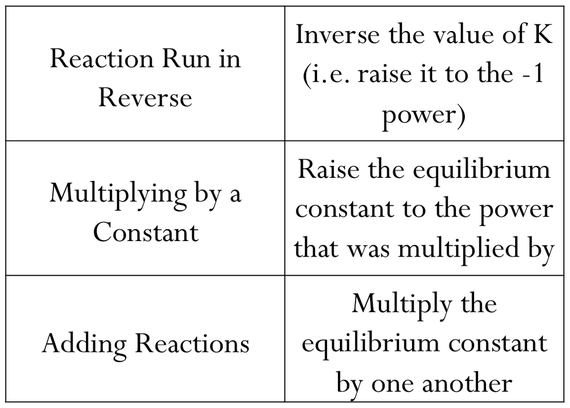
Flipping reactions is essentially the equivalent of saying “let’s start with the products and end with the reactants”. Because of this, we can find out what our equilibrium constant will be by writing out the formula for the equilibrium constant for both equations:

If our original reaction was A ⇌ B, that would make K = [B]/[A]. Let’s think about our K value for the reverse, B ⇌ A. You may think that the equilibrium constant would be the same because A ⇌ B is reversible, but in this case the forward reaction is what what the backwards reaction. Rewriting our equilibrium formula for B ⇌ A we find that K2 = [A]/[B] = 1/K. 

From here we can conclude that flipping a reaction gives it a K value of 1/K. Let’s look at an example:

Because the reaction we’re told to find K for is just the reverse of the reaction, we can find K for 2NH3 ⇌ N2 + 3H2 to be 1/0.118 = 8.47.

Let’s take a look at what happens when we multiply a reaction by a coefficient. When we say multiplying a reaction we mean taking something like A ⇌ B and multiplying it by a constant n to form nA ⇌ nB (ex. If n=2 we would have 2A ⇌ 2B). This does not change the reaction besides the moles that go in and out (the equation remains balanced however!), but it does change the equilibrium constant. Let’s explore why:

For the reaction A ⇌ B, K = [B]/[A]. We established this same fact in part 1.

However, for the reaction 2A ⇌ 2B, K = [B]^2 / [A]^2 = ([B]/[A])^2 = K^2.

Let’s see if we can generalize this to any n coefficient:

For the general multiplied reaction nA ⇌ nB, K = [B]^n / [A]^n = ([B]/[A])^n = K^n.

What does this tell us about how multiplying a reaction impacts the equilibrium constant? It tells us that when we multiply a reaction, our K value gets exponentiated that same amount. For example, multiplying a reaction by 3 cubes K. Let’s take a look at a real example:

Like before, Consider the reaction N2 + 3H2 ⇌ 2NH3 that has a K = 0.118.

Find the equilibrium constant for the reaction (1/2)N2 + (3/2)H2 ⇌ NH3.

In this case, we've multiplied our reaction by ½. This means that our K value, 0.118, is raised to the ½ power. K = (0.118)^(½) = 0.343.

The crux of this section is what happens when we learn what happens when reactions are added together. Like before, let’s look at a general example without any real chemicals:

A ⇌ B : K = [B]/[A]

C ⇌ D : K = [D]/[C]

A + C ⇌ B + D : K = [B][D] / [A][C].

What does this mean? We have two reactions, A ⇌ B and C ⇌ D that we’re adding together to form A + C ⇌ B + D. However, take a look at what happens to our equilibrium constants. It may seem like nothing happened, but in fact in adding the reactions together we actually multiplied our Ks! K1 was [B]/[A] and K2 was [D]/[C] which multiplied to form [B][D] / [A][C]! Therefore, when we add reactions together we multiply together the K values. 

We’ve covered 3 main rules that you may have to combine in problems (we’ll cover that next!). Here’s a table of the rules we’ve learned so far:

Reaction 1: (1/2)N2 + (1/2)O2 ⇌ NO …. K = 6.55 * 10^(-13)

Reaction 2: 2NO + O2 ⇌ 2NO2 …. K = 6.9 * 10^(5)

We have two reactions that sorta look like the reaction we want so let’s think about ways to get these reactions into a form that we can add them together to form our reaction.

If we multiply reaction 1 by 2, we’ll get N2 + O2 ⇌ 2NO and the subsequent K value of (6.55 * 10^(-13))^2 = 4.3 * 10^(-25).

Next, adding together our multiplied reaction 1 with reaction 2 we’ll find that they equal our reaction that we want! The NOs will cancel out, the (1/2)N2 is now N2, and our O2s will add to form 2O2. Therefore, our final K will be 4.3 * 10^(-25) * 6.9 * 10^(5) = 3.0 * 10^(-19).

You may be wondering how to know what to do when you’re given one of these problems without any clear steps. There really is no rhyme or reason and as long as you follow the rules, but in general, PRACTICE MAKES PERFECT! Practicing these problems will give you a better chemistry spidey-sense and realize when to flip a reaction, when to multiply a reaction, and when to add them together. You got this!